Enhanced diffusion in dense needle liquids
Felix Höfling, Erwin Frey, Thomas Franosch
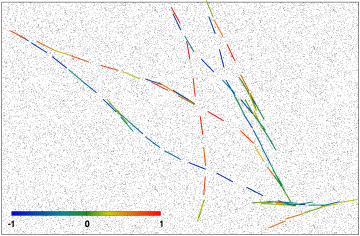 Dense liquids of infinitely thin, hard needles challenge Statistical Mechanics: while its equilibrium properties correspond to those of an ideal gas, the steric hindrance of the needles induces complex dynamic behavior. An intriguing phenomenon is the enhancement of translational diffusion over several orders of magnitude as density increases. Such a behavior contradicts the experience that transport becomes slow in dense complex liquids. We observed the emergence of a spacious zigzag motion of the needle (see movie), which results in a power-law increase of the diffusion coefficient with the mysterious exponent 0.8.
Dense liquids of infinitely thin, hard needles challenge Statistical Mechanics: while its equilibrium properties correspond to those of an ideal gas, the steric hindrance of the needles induces complex dynamic behavior. An intriguing phenomenon is the enhancement of translational diffusion over several orders of magnitude as density increases. Such a behavior contradicts the experience that transport becomes slow in dense complex liquids. We observed the emergence of a spacious zigzag motion of the needle (see movie), which results in a power-law increase of the diffusion coefficient with the mysterious exponent 0.8.
The zigzag motion can be explained by the persistence of longitudinal momentum, which becomes apparent in a two-step decay of the velocity-autocorrelation function. Via a Green-Kubo relation, the "persistence time" is proportional to the diffusion coefficient and diverges with the same power law. Although the exponent of the power law is not yet fully rationalized, we provide simple scaling arguments to capture the essential mechanism of the zigzag motion.

