Coexistence and survival in conservative Lotka-Volterra systems
Johannes Knebel, Torben Krüger, Markus F. Weber, Erwin Frey
Understanding the stability of ecological networks is of pivotal importance in theoretical biology. An intriguing question is how coexistence and extinction of species depend on the interaction network between species. Is it the topology of the network that sets the level of biodiversity? And how important is the strength of a single interaction link? One paradigm to address these biologically relevant questions from a theoretical point of view is the conservative Lotka-Volterra system. The role of this model in the class of general Lotka-Volterra systems can be compared to the relevance of the harmonic oscillator in the class of general oscillators.
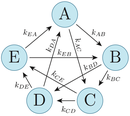
In this Letter, we introduce a general classification of coexistence scenarios in conservative Lotka-Volterra networks with an arbitrary number of species. We elucidate the consequences of the interplay between the network structure and the strengths of its interaction links on global stability. By employing the algebraic concept of the Pfaffian, we are able to quantify the extinction process. We illustrate our findings for systems of four and five species and find a generalized scaling law for the extinction time in finite systems.